four significant digits|Significant figures : Cebu Step 3: Now, count the number of digits after the first number from the given . Viaja fácilmente desde Aeropuerto Las Vegas (LAS) a Excalibur Hotel and Casino con Rome2Rio. Rome2Rio es un motor de información para organizar viajes de puerta a puerta y hacer reservas, que te ayuda a llegar desde y hasta cualquier lugar del mundo" Encuentra aquí todas las opciones de transporte para tu viaje desde Aeropuerto Las Vegas (LAS) .
four significant digits,Definition. The significant figures of a given number are those significant or important digits, which convey the meaning according to its accuracy. For example, 6.658 has four significant digits. These substantial figures provide precision to the numbers. They .
Step 3: Now, count the number of digits after the first number from the given . Round a number to a quantity of significant figures that you provide. Enter whole numbers, real numbers, scientific notation or e notation. Example inputs are, 3500, 35.0056, 3.5 x 10^3 and 3.5e3.Significant figures Our significant figures calculator works in two modes – it performs .The number of significant digits can be identified by counting all the values starting from the 1st non-zero digit located on the left. These numbers are reliable and necessary to indicate the quantity of a length, volume, .
Significant figures (or significant digits) are the number of digits important to determine the accuracy and precision of measurement, such as length, mass, or volume. Significant digits in math convey the value of a number .
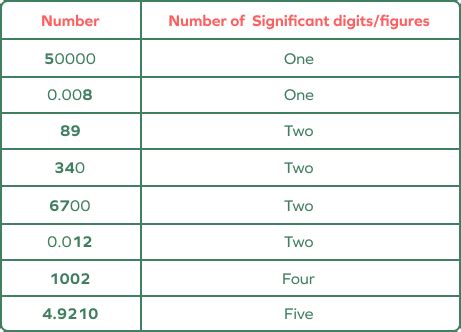
four significant digits1.1 has two significant figures (1, 1). 13.55 has four significant figures (1, 3, 5, 5). Rule 2 - any zero contained between two non-zero numbers is significant. If a zero forms part of a string of digits AND falls between . In this example you would want to enter 2.00 for the multiplier constant so that it has the same number of significant figures as the radius entry. The resulting answer would be 4.70 which has 3 significant figures. Related Calculators. To learn more about rounding significant figures see our Rounding Significant Figures Calculator.652.1 miles $- 4$ significant figures. 3.4 inches $- 2$ significant figures. (2) All zeros in between non-zero digits are significant. 23.0041 $- 6$ significant digits. 4005 feet $- 4$ significant digits. (3) Zeros on the .

12. four significant figures. As mentioned above, we use an overbar to indicate when a zero that looks insignificant is actually significant. For example, the precision [2] of \(7,400\) is the hundreds place; if we rounded anything from \(7,350\) to \(7,449\) to the nearest hundred, we would write the result as \(7,400\). An overbar shows that .
four significant digits Significant figures 12. four significant figures. As mentioned above, we use an overbar to indicate when a zero that looks insignificant is actually significant. For example, the precision [2] of \(7,400\) is the hundreds place; if we rounded anything from \(7,350\) to \(7,449\) to the nearest hundred, we would write the result as \(7,400\). An overbar shows that . In this example, 5.544 has four significant digits, and 2.2 has two significant digits. When subtracting the numbers on a calculator, the value is 3.344. However, because 2.2 only has two .
2.437 includes four significant figures; 327 includes three significant figures. 2) When zeros are between digits that are not zeros, they are significant. For example: 700021 includes six significant figures; 3049 includes four significant figures. 3) When a zero is to the left of the first digit that is not a zero, it is not significant. For . When a number is expressed in scientific notation, the number of significant digits (or significant figures) is the number of digits needed to express the number to within the uncertainty of calculation. For example, if a quantity is known to be 1.234+/-0.002, four figures would be significant The number of significant figures of .
@ionelberdin that is incorrect. I.e. from the article you linked: "Zeros to the right of the last non-zero digit (trailing zeros) in a number with the decimal point are significant". The number 4.2 to three significant figures is 4.20. – Significant Figures Rules. Non-zero digits are always significant; Zeros between non-zero digits are always significant; Leading zeros are never significant; Trailing zeros are only significant if the number contains a decimal point; Related Calculators. For math with significant figures see our Significant Figures Calculator.
Identify the Significant Figures. First, identify the significant figures in the number you wish to round. Significant figures are all digits except leading zeros. For example, in the number 0.00234, there are three significant figures (2, 3, and 4). Rule 2: Determine the Rounding Digit. The rounding digit is the first non-significant figure.Rule # 01: "Remember that every digit that is not zero is significant" For Example: If you input 2.547 in this significant figures calculator, it will show you four (4) sig figs in it; Likewise, 427 has three significantYou simply include all the significant figures in the leading number. For example, the number 450 has two significant figures and would be written in scientific notation as 4.5 × 10 2, whereas 450.0 has four significant figures and would be written as 4.500 × 10 2. In scientific notation, all significant figures are listed explicitly.The significant figures of a number are those digits that carry meaning contributing to its precision. Thus the number of significant digits depends on the least count of the measuring instrument. All the certain digits and .
有效数字(significant figures,significant digits,简写 sig figs),其代表一个數是由若干位數字组成,其中影响其测量精度的数字被称作有效数字,也称有效数位 [1] 。. 有效数字指科学计算中用以表示一定长度浮点数精度的那些数字。一般指一个用小数形式表示的浮点数中,从第一个非零的数字算起的 . All zeros between other significant digits are significant. The number of significant figures is determined by starting with the leftmost non-zero digit. The leftmost non-zero digit is sometimes called the most significant digit or the most significant figure. For example, in the number 0.004205, the '4' is the most significant figure.To avoid uncertainty, use scientific notation to place significant zeroes behind a decimal point: \(8.200 \times 10^3\) has four significant digits \(8.20 \times 10^3\) has three significant digits \(8.2 \times 10^3 \) has two significant digits Significant Digits in Multiplication, Division, Trig. functions, etc.

Rounding 15.9375 to 4 significant figures, we get: The last digit we keep is 3: 15.9375. The digit after 3 is 7. 7 is greater than 5. Therefore, the last digit should increase by 1: 3 + 1 = 4. The rounded number will be 15.94. This means that if you pay for the dress with 20 dollars, you will get $(20 - 15.94) = $4.06 as change.So now back to the example posed in the Rounding Tutorial: Round 1000.3 to four significant figures. 1000.3 has five significant figures (the zeros are between non-zero digits 1 and 3, so by rule 2 above, they are significant.) We need to drop the final 3, and since 3 < 5, we leave the last zero alone. .
four significant digits|Significant figures
PH0 · Significant figures
PH1 · Significant Figures: Definition, Examples, Rules,
PH2 · Significant Figures
PH3 · Sig Fig Calculator
PH4 · Rounding Significant Figures Calculator